Our member Eszter Fehér successfully defended her PhD thesis Wrinkling behavior of highly stretched thin films.
Brief summary: In the literature of the wrinkling of ultrathin films, theoretical models were often used to investigate phenomena that fall outside the range of validity of their assumptions. Contradictory results were published regarding the wrinkling of axially stretched, clamped, rectangular films. Based on the Föppl-von Kármán plate theory (FvK) wrin- kles appear on the surface and their amplitude scales with the macroscopic strain. A finite membrane strain extension of the FvK theory (eFvK) predicted that only a bounded region of the macroscopic strain and aspect ratio parameters exhibit wrinkling in the problem.
The first goal of the work is to verify these predictions experimentally. Secondly, the eFvK model was extended into directions, that are beneficial in the modeling of real-life structures. The effect of material and geometrical properties and nonlinearities on wrinkling was also examined in detail.
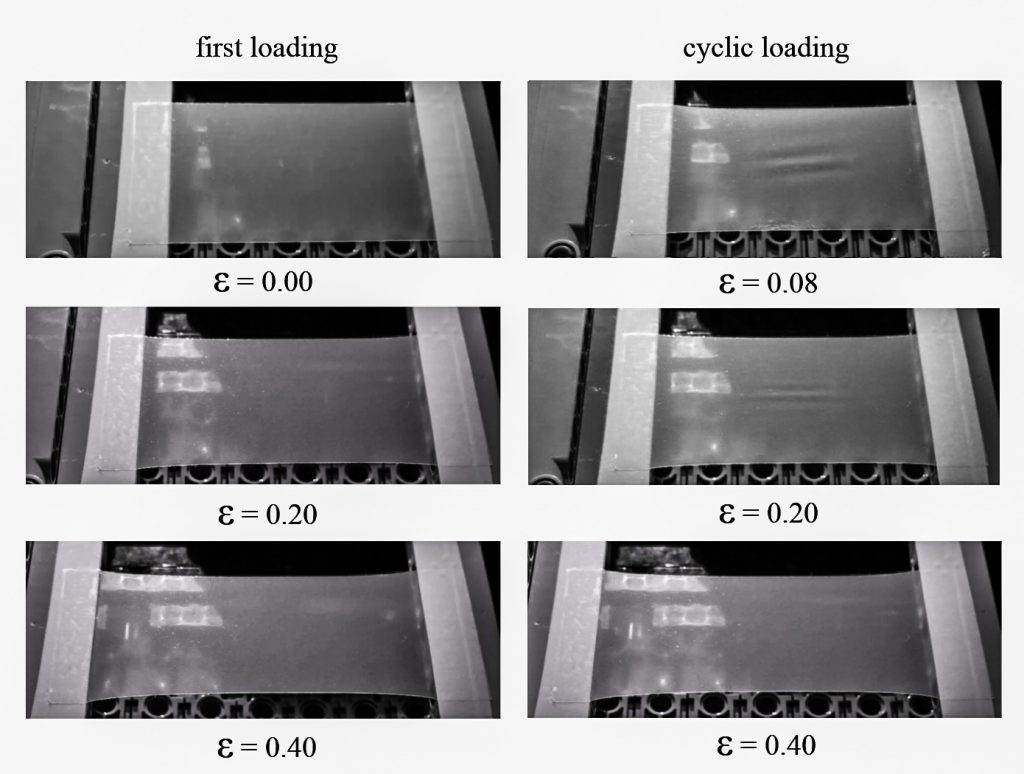
Orthotropy was incorporated into the model and its effect was investigated. By comparing the results of the orthotropic model and experiments carried out on pre- stressed polyurethane films, the predictions of the eFvK model were experimentally verified. Motivated by further experimental observations, a pseudoelastic model based on the Mullins effect was also proposed and it was shown, that it can explain the intriguing phenomenon arisen during the prestressing of polyurethane films.
The eFvK model was extended to curved surfaces, and it was shown, that the intrinsic curvature can reduce wrinkling. Finally, we carried out an investigation of the parameter space by letting the thickness of the film arbitrary small. We introduced the concept of disturbed zones near the clamps which gave a physical explanation of the aspect ratio dependency of the wrinkling in the model problem.In the literature of the wrinkling of ultrathin films, theoretical models were often used to investigate phenomena that fall outside the range of validity of their assumptions. Contradictory results were published regarding the wrinkling of axially stretched, clamped, rectangular films. Based on the Föppl-von Kármán plate theory (FvK) wrin- kles appear on the surface and their amplitude scales with the macroscopic strain. A finite membrane strain extension of the FvK theory (eFvK) predicted that only a bounded region of the macroscopic strain and aspect ratio parameters exhibit wrinkling in the problem.
The first goal of the work is to verify these predictions experimentally. Secondly, the eFvK model was extended into directions, that are beneficial in the modeling of real-life structures. The effect of material and geometrical properties and nonlineari- ties on wrinkling was also examined in detail.
Orthotropy was incorporated into the model and its effect was investigated. By comparing the results of the orthotropic model and experiments carried out on prestressed polyurethane films, the predictions of the eFvK model were experimentally verified. Motivated by further experimental observations, a pseudoelastic model based on the Mullins effect was also proposed and it was shown, that it can explain the intriguing phenomenon arisen during the prestressing of polyurethane films.
The eFvK model was extended to curved surfaces, and it was shown, that the intrinsic curvature can reduce wrinkling. Finally, we carried out an investigation of the parameter space by letting the thickness of the film arbitrary small. We introduced the concept of disturbed zones near the clamps which gave a physical explanation of the aspect ratio dependency of the wrinkling in the model problem.